Let me just start with this.  If you live in Georgia, say within a 2 hour drive to the UGA Griffin campus, seriously consider joining the Masters’ or EdS program.  I’m in my first semester.  It’s amazing!  ‘Nuff said.

Maybe it’s just me… I thought I understood everything I needed to know about fraction equivalence… until this week.  If you get to the end and think, “Oh, I already knew that!” I apologize.  This is post is really for me to reflect a bit.  If it helps anyone else make sense of fractions…well that’s just gravy!

It all started with an assignment for one of my graduate classes. The assignment was to read Chapter 3 from Number Talks Fractions, Decimals, and Percents by Sherry Parrish and reflect on one of the big ideas and the common misconceptions connected to those big ideas.  I chose to reflect on fraction equivalence.

In the section on equivalence, Dr. Parrish talks about how students want to take fractions like 1/4 and multiply by two to get an equivalent fraction of 2/8. This misconception may be fostered by teachers who wish to make equivalent fractions easy for their students to remember. This is never a good idea!  Because really… if you multiply 1/4 by two, that means you have 2 groups of 1/4.  And 2 groups of 1/4 gives you 2/4 and 1/4 can’t be the same as 2/4.

What I learned next came from a phone conversation I had with Graham Fletcher about 15 seconds after I finished reading the chapter.  Sometimes I just think he knows when I’m learning some math and gives me a call.  He had a question about equivalent fractions. Over the course of about 45 minutes talking on the phone, I think we both deepened our understandings about what makes two fractions equivalent.

Take the rule of multiplying the numerator and the denominator both by the same number to make an equivalent fraction.  If we look at 1/4 and multiply the numerator and denominator by two to get 2/8, we get an equivalent fraction, but this isn’t necessarily the whole story.  To really understand fraction equivalence, I had to be asked to dive a little deeper. Graham asked me to dive deeper.  As we talked, multiplying by one came up, then the multiplicative identity.  These ideas definitely strengthened my understanding of fraction equivalence.

I thought I now had a deep understanding of fraction equivalence.  But wait, there’s more.  This is the best part.  I went to class this past Saturday and Dr. Robyn Ovrick gave us this:

We were asked to fold the paper as many times as we wanted as long as all of the sections were the same size.  Some of us folded once (guilty – I hate folding almost as much as I hate cutting).  We shared our folds and Robyn recorded what several of us did on the smart board.  Then she asked what we noticed.  This is where everything came together for me.  I tried to share my thoughts but I don’t think I was very successful.  I was really excited about this.  Here is my (1 fold) representation of an equivalent fraction for 1/4:

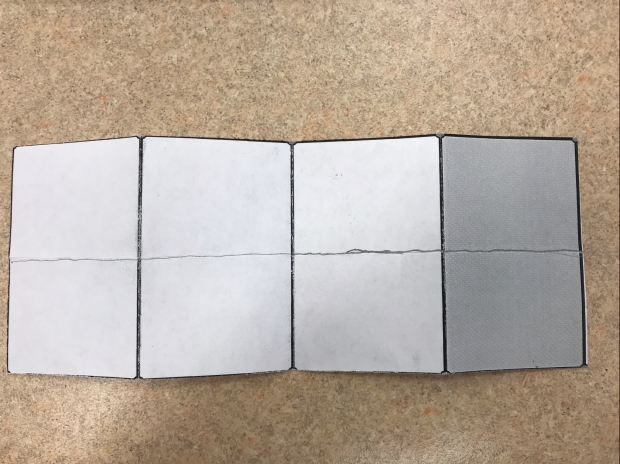
For my example, someone said the number of pieces doubled, and at this point (my eyes probably almost shot out of my head) I thought, but the size of the pieces are half as big.  I’m usually pretty reserved and quiet, but this was too much.  So, with a lot of help from colleagues in class who know me a bit better than the others it all came clear to me.  We visually made equivalent fractions, but connected the visual to the multiplicative identity and even explained it in the context of paper folding.

Here it is.

The original paper shows 1/4.  When we fold it in half horizontally, we get 2 times as many pieces and the pieces are half the size.  This can be represented here:

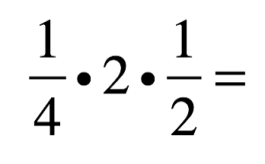
The 1/4 represents the original fraction. The 2 shows that we got twice as many pieces, and the 1/2 shows that each of those pieces is half the size.  With a little multiplication and the commutative property we can get something that looks like this:

Knowing that two halves is one whole is definitely part of this understanding, but seeing where it can come from in the context of paper folding allows an opportunity for a much deeper understanding. The numerator tells that there are twice as many sections as before and the denominator (really the fraction 1/2) says that the pieces are now half the size.  We looked at another example of how someone folded 1/4 (someone who folded 8 times!) and noticed that it worked the similarly – we got 8 times as many pieces and the pieces were each 1/8 the size of the original.  I don’t think anyone thought it wouldn’t work similarly, but it sure is nice to see your ideas validate something you thought you really understood before waking up that morning!

I’m still thinking about this and I keep making more connections.  This morning, in a place where I think I do my best thinking (the shower!), I realized that this is connected to the strategy of doubling and halving for multiplication.  I’ll leave you with that.  Time for you to chew.